借助networkx提供的节点、边、图绘制便捷功能,自己写了一个Prim逻辑实现,练手。
最小生成树现实中场景:比如大城市多个地点之间修建连通的公路,如何选择连接的点,才使得建造代价最小、且能够连通所有城市地点。
Prim Algorithm核心是:迭代寻找最小权边的点,接入图。
把图中的节点分为U,V两部分,可以先把V装满图中的点,V=[v2,v3,v4,v5,,,vn]。算法启动时候,U=[]为空,任取一点假设是v1装入U,U=[v1],然后将V中所有与U中点邻接的点形成的边,取权最小的,把点从V中移除放入U。依次迭代,注意,越往后,U中点越多,要计算所有U中点和V中点邻接的边,取最小的权。
import sys
import networkx as nx
import matplotlib.pyplot as plt
# 这里实现的代码里面有两个潜在的遗留问题未解决:
# 1,选边时候,对有可能形成的环未做处理。
# 2,加点时候,如果出现多条相同权边的选择,算法是否会稳定?
def prim(G):
my_edge_bfs = list(nx.edge_bfs(G=G, source=G.nodes))
print('所有联通子路径', my_edge_bfs)
print('所有边', G.edges())
print('所有边权', G.edges(data=True))
nodes = list(G.nodes)
U = [nodes[0]]
V = nodes[1:]
min_edges = []
while True:
if len(V) == 0:
break
print('-----')
print('节点划分', U, V)
node_visit = []
for vn in V:
for un in U:
for med in my_edge_bfs:
if (un in med) and (vn in med):
ws = get_edge_weight(med, G)
print('存在通路->', med, ws)
node_visit.append((med, ws))
print('访问节点放入列表', node_visit)
min_edge = find_min_edge(node_visit)
print('通路的最小权边', min_edge)
min_edges.append(min_edge)
for e in min_edge[0]:
if e not in U:
U.append(e)
# 找出两个list的交集
c = list(set(min_edge[0]).intersection(set(V)))
print('V中应该删掉的节点:', c[0])
V.remove(c[0])
print('*最小生成树=', U)
print('*最小生成树的边=', min_edges)
return min_edges
def my_graph():
# 构造一个有权边的无向图,然后找出最小生成树
G = nx.Graph() # 无向图
nodes = ['a', 'b', 'c', 'd', 'e', 'f']
G.add_nodes_from(nodes)
G.add_edges_from([('a', 'b', {'weight': 6}),
('a', 'c', {'weight': 1}),
('a', 'd', {'weight': 5}),
('b', 'c', {'weight': 5}),
('c', 'd', {'weight': 5}),
('b', 'e', {'weight': 3}),
('c', 'e', {'weight': 6}),
('e', 'f', {'weight': 6}),
('c', 'f', {'weight': 4}),
('d', 'f', {'weight': 2})])
pos = nx.spring_layout(G)
nx.draw(G, pos,
node_color='green',
node_size=500,
font_size=10,
font_color='black',
edge_color='gray',
width=1,
alpha=0.5,
with_labels=True)
my_edge_labels = nx.get_edge_attributes(G, 'weight')
nx.draw_networkx_edge_labels(G, pos, edge_labels=my_edge_labels)
min_edges = prim(G)
edge_list = []
for edge in min_edges:
edge_list.append(edge[0])
# 把最小生成树的边着色加粗重新描边
nx.draw_networkx_edges(G, pos,
edgelist=edge_list,
width=8,
alpha=0.5,
edge_color="r")
plt.show()
# 传入一个边,找到该边对应的权
# 这里没有解决如果若干条边同时具有相同的权值,应该返回一个列表
def get_edge_weight(edge, G):
WEIGHT = sys.maxsize
weights = nx.get_edge_attributes(G, 'weight')
for e in G.edges():
if e == edge:
w = weights.get(e)
WEIGHT = w
return WEIGHT
# 这里没有解决如果若干条边同时具有相同的权值问题,应该返回一个列表,装入所有相同值的边
def find_min_edge(edges):
w_val = []
for e in edges:
w_val.append(e[1])
min_w = min(w_val)
i = w_val.index(min_w)
return edges[i]
if __name__ == '__main__':
my_graph()
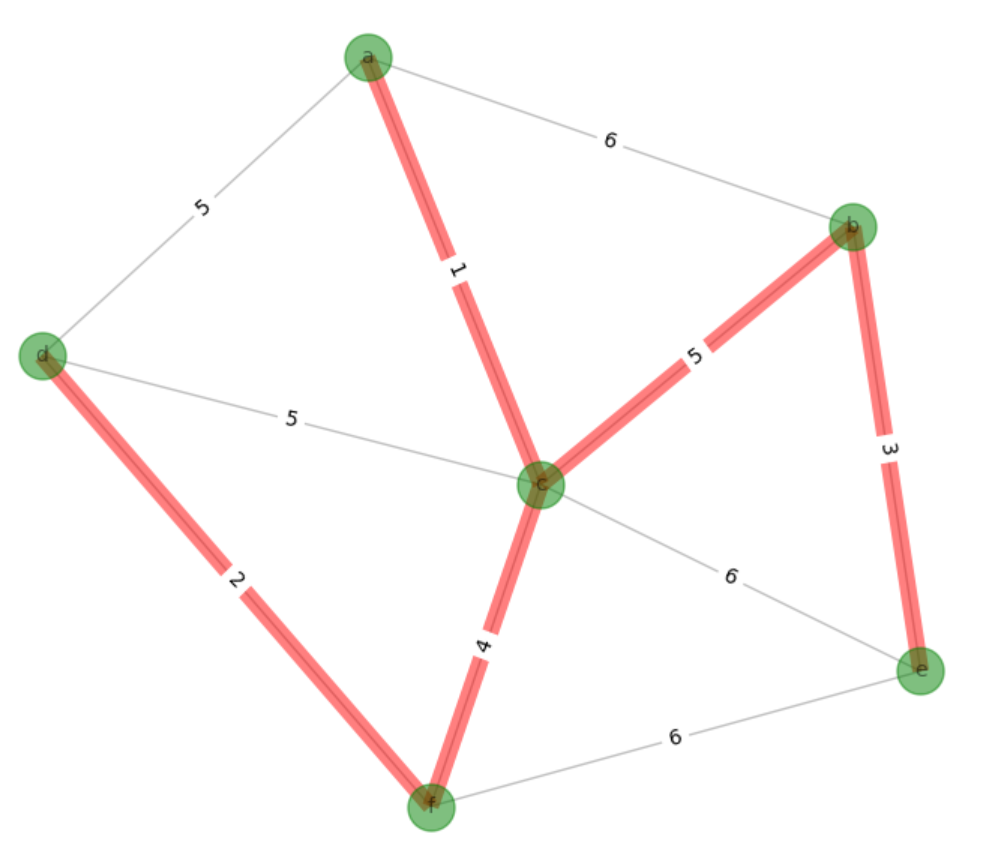
生成的最小生成树图,红色权边即为:
运行日志:
所有联通子路径 [('a', 'b'), ('a', 'c'), ('a', 'd'), ('b', 'c'), ('b', 'e'), ('c', 'd'), ('c', 'e'), ('c', 'f'), ('d', 'f'), ('e', 'f')]
所有边 [('a', 'b'), ('a', 'c'), ('a', 'd'), ('b', 'c'), ('b', 'e'), ('c', 'd'), ('c', 'e'), ('c', 'f'), ('d', 'f'), ('e', 'f')]
所有边权 [('a', 'b', {'weight': 6}), ('a', 'c', {'weight': 1}), ('a', 'd', {'weight': 5}), ('b', 'c', {'weight': 5}), ('b', 'e', {'weight': 3}), ('c', 'd', {'weight': 5}), ('c', 'e', {'weight': 6}), ('c', 'f', {'weight': 4}), ('d', 'f', {'weight': 2}), ('e', 'f', {'weight': 6})]
-----
节点划分 ['a'] ['b', 'c', 'd', 'e', 'f']
存在通路-> ('a', 'b') 6
存在通路-> ('a', 'c') 1
存在通路-> ('a', 'd') 5
访问节点放入列表 [(('a', 'b'), 6), (('a', 'c'), 1), (('a', 'd'), 5)]
通路的最小权边 (('a', 'c'), 1)
V中应该删掉的节点: c
*最小生成树= ['a', 'c']
*最小生成树的边= [(('a', 'c'), 1)]
-----
节点划分 ['a', 'c'] ['b', 'd', 'e', 'f']
存在通路-> ('a', 'b') 6
存在通路-> ('b', 'c') 5
存在通路-> ('a', 'd') 5
存在通路-> ('c', 'd') 5
存在通路-> ('c', 'e') 6
存在通路-> ('c', 'f') 4
访问节点放入列表 [(('a', 'b'), 6), (('b', 'c'), 5), (('a', 'd'), 5), (('c', 'd'), 5), (('c', 'e'), 6), (('c', 'f'), 4)]
通路的最小权边 (('c', 'f'), 4)
V中应该删掉的节点: f
*最小生成树= ['a', 'c', 'f']
*最小生成树的边= [(('a', 'c'), 1), (('c', 'f'), 4)]
-----
节点划分 ['a', 'c', 'f'] ['b', 'd', 'e']
存在通路-> ('a', 'b') 6
存在通路-> ('b', 'c') 5
存在通路-> ('a', 'd') 5
存在通路-> ('c', 'd') 5
存在通路-> ('d', 'f') 2
存在通路-> ('c', 'e') 6
存在通路-> ('e', 'f') 6
访问节点放入列表 [(('a', 'b'), 6), (('b', 'c'), 5), (('a', 'd'), 5), (('c', 'd'), 5), (('d', 'f'), 2), (('c', 'e'), 6), (('e', 'f'), 6)]
通路的最小权边 (('d', 'f'), 2)
V中应该删掉的节点: d
*最小生成树= ['a', 'c', 'f', 'd']
*最小生成树的边= [(('a', 'c'), 1), (('c', 'f'), 4), (('d', 'f'), 2)]
-----
节点划分 ['a', 'c', 'f', 'd'] ['b', 'e']
存在通路-> ('a', 'b') 6
存在通路-> ('b', 'c') 5
存在通路-> ('c', 'e') 6
存在通路-> ('e', 'f') 6
访问节点放入列表 [(('a', 'b'), 6), (('b', 'c'), 5), (('c', 'e'), 6), (('e', 'f'), 6)]
通路的最小权边 (('b', 'c'), 5)
V中应该删掉的节点: b
*最小生成树= ['a', 'c', 'f', 'd', 'b']
*最小生成树的边= [(('a', 'c'), 1), (('c', 'f'), 4), (('d', 'f'), 2), (('b', 'c'), 5)]
-----
节点划分 ['a', 'c', 'f', 'd', 'b'] ['e']
存在通路-> ('c', 'e') 6
存在通路-> ('e', 'f') 6
存在通路-> ('b', 'e') 3
访问节点放入列表 [(('c', 'e'), 6), (('e', 'f'), 6), (('b', 'e'), 3)]
通路的最小权边 (('b', 'e'), 3)
V中应该删掉的节点: e
*最小生成树= ['a', 'c', 'f', 'd', 'b', 'e']
*最小生成树的边= [(('a', 'c'), 1), (('c', 'f'), 4), (('d', 'f'), 2), (('b', 'c'), 5), (('b', 'e'), 3)]

